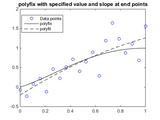
polyfix(x,y,n,xfix,yfix,xder,dydx)
Fit polynomial p to data, but match exactly at one or more points
You are now following this Submission
- You will see updates in your followed content feed
- You may receive emails, depending on your communication preferences
Editor's Note: This file was selected as MATLAB Central Pick of the Week
Sometimes we want to fit a polynomial to some data, but want to force a perfect match to some known values at one or more points. This function returns such a polynomial.
Cite As
Are Mjaavatten (2026). polyfix(x,y,n,xfix,yfix,xder,dydx) (https://www.mathworks.com/matlabcentral/fileexchange/54207-polyfix-x-y-n-xfix-yfix-xder-dydx), MATLAB Central File Exchange. Retrieved .
Acknowledgements
Inspired: Bayesian Inference from Hans Bathe
General Information
- Version 1.3.1.2 (2.66 KB)
MATLAB Release Compatibility
- Compatible with any release
Platform Compatibility
- Windows
- macOS
- Linux
| Version | Published | Release Notes | Action |
|---|---|---|---|
| 1.3.1.2 | Changed image |
||
| 1.3.1.1 | Added image |
||
| 1.3.1.0 | Fixed the error in version 1.3.0.0 pointed out by Jacob Bresler. |
||
| 1.3.0.0 | As requested by Jacob Bresler, I have added optional outputs S and mu, to make the output from polyfix conform with polyfit |
||
| 1.2.0.0 | Fixed trivial errors in the help section |
||
| 1.1.0.0 | Version 1.1 includes an option for specifying derivatives. p is now a row vector, for consistency with polyfit |
||
| 1.0.0.0 |
