bicgstabl
Solve system of linear equations — stabilized biconjugate gradients (l) method
Syntax
Description
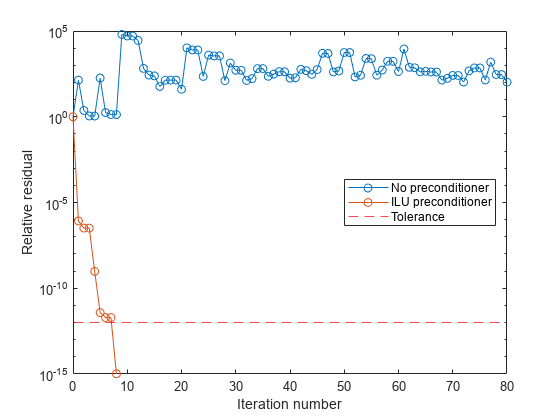
x = bicgstabl(A,b)A*x = b for
x using the Biconjugate Gradients Stabilized (l) Method. When the attempt is
successful, bicgstabl displays a message to confirm convergence. If
bicgstabl fails to converge after the maximum number of iterations or
halts for any reason, it displays a diagnostic message that includes the relative residual
norm(b-A*x)/norm(b) and the iteration number at which the method
stopped.
[
returns a flag that specifies whether the algorithm successfully converged. When
x,flag] = bicgstabl(___)flag = 0, convergence was successful. You can use this output syntax
with any of the previous input argument combinations. When you specify the
flag output, bicgstabl does not display any
diagnostic messages.
Examples
Input Arguments
Output Arguments
More About
Tips
Convergence of most iterative methods depends on the condition number of the coefficient matrix,
cond(A). WhenAis square, you can useequilibrateto improve its condition number, and on its own this makes it easier for most iterative solvers to converge. However, usingequilibratealso leads to better quality preconditioner matrices when you subsequently factor the equilibrated matrixB = R*P*A*C.You can use matrix reordering functions such as
dissectandsymrcmto permute the rows and columns of the coefficient matrix and minimize the number of nonzeros when the coefficient matrix is factored to generate a preconditioner. This can reduce the memory and time required to subsequently solve the preconditioned linear system.
References
[1] Barrett, R., M. Berry, T. F. Chan, et al., Templates for the Solution of Linear Systems: Building Blocks for Iterative Methods, SIAM, Philadelphia, 1994.
Extended Capabilities
Version History
Introduced before R2006a